At relative positions between two geometric figures constitute the study of the possibilities of interaction between these elements in the space in which they occupy. In other words, the figures are classified according to number or how interactions between them occur. Trivial relative positions, for example, take place between point and straight, which are just two: a point belongs to a line or does not belong to it.
Relative positions between two lines
1 – parallel lines: Two lines are parallel when they don't have Score in common. Remembering that this is true for the entire length of these lines and that they are infinite.
2 – straightcompetitors: Two lines are concurrent when they have a single point in common. When the angle formed between these two lines is 90°, we say that they are perpendicular.
3 – straightcoincident: Two lines are coincident when they have two or more points in common. It is possible to show that if the lines r and s have two (or more) points in common, then r = s. Therefore, coincident lines are seen as a single line, or as two distinct lines that occupy the same space.
Relative positions between straight and plane
1 – straightandflatparallels: a line is parallel to a flat when they have no common ground.
2 – straightand competing plan: a line r is concurrent with an α plane when they have a single Score P in common. If by P passes at least two straight distinct lines contained in plane α, each perpendicular to line r, then line r is perpendicular to plane α.
3 – straightcontainedat theflat: a line is contained in a plane when all its points are also points on the plane.
Relative positions between planes
1 – plansparallels: two planes are parallel when there is no meeting point between them.
2 – planscompetitors: two planes are concurrent when they intersect. The intersection between two planes is equal to a straight line.
3 – planscoincident: Two planes are coincident when all foreground points are also background points.
The following image shows the intersection of two concurrent planes.
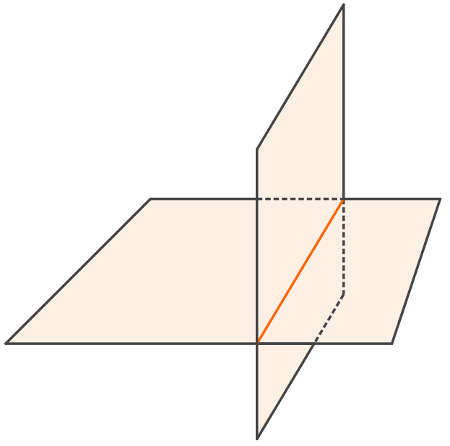
two planes are perpendicular when one of them contains a straight line perpendicular to the other plane.
Relative positions between a point and a circle
given one circumference c, with center O and radius r, and a point P, we will have the following relative positions:
1 – Pointinternal: point P belongs to the inner region of the circumference whenever the distance between P and the center O of the circle is smaller than the radius r. In other words, wheneverOP < r.
2 – Pointbelongingàcircumference: point P belongs to circle c whenever dOP = r.
3 – outside point: a point P belongs to the outer region of the circle c whenever dOP > a.
Relative positions between straight and circle
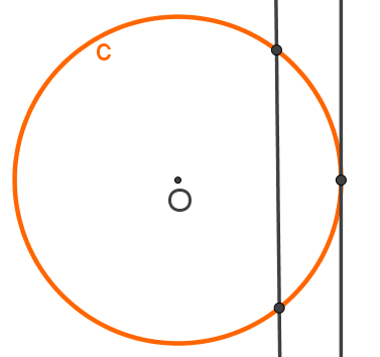
1 – straightexternal: the line and the circle have no point in common.
2 – straighttangent: the line and the circle have only one point in common.
3 – straightdrying: the line and the circle have two points in common.
The following image shows what a tangent line and a secant line to the circle look like.
Relative positions between two circles
1 – Disjoint Circumferences
The) Disjointinternal: the circles have no point in common, and all the points of one of them are in the interior region of the other.
B) Disjointexternal: The circles have no point in common, and all the points of one of them are on the outer region of the other.
2 – Tangent Circumferences
The) Tangentsinternal: the circles have only one point in common and all the other points of one of them are in the inner region of the other.
B) Tangentsexternal: the circles have only one point in common and all the other points of one of them are in the outer region of the other.
3 – Circumferencesdrying: circles have two points in common.
By Luiz Paulo Moreira
Graduated in Mathematics
Source: Brazil School - https://brasilescola.uol.com.br/o-que-e/matematica/o-que-sao-posicoes-relativas.htm
