Dilationthermal it is a physical phenomenon arising from an increase in the temperature of a body. When a body is exposed to some source of heat, your temperature it can undergo variations, increasing the agitation of the molecules, which oscillate around a larger space.
This microscopic variation in the vibration of molecules can be perceived on a macroscopic scale, as when an iron bar stays slightly larger as a result of heating.
linear dilation
Dilationlinear of solids is the physical phenomenon that occurs when linearly shaped bodies that are in solid state, such as wires, cables, needles, bars, pipes, undergo a variation in temperature. To calculate the magnitude of the linear dilation, we use the coefficientindilationlinear of material.
Examples of linear thermal expansion
Warping of train tracks due to the large thermal amplitude during day and night cycles. Because of this effect, the expansion joint is used, a small space between two consecutive bars.
The copper wires used in the transmission of electrical current on poles are always greater than the distance between the poles. If they weren't, on cold days, these conductors would suffer negative variations in their length, and may suffer ruptures
superficial dilation
Dilationshallow of solids is the variation in the area of a body that is in the solid state due to an increase in its temperature. The calculation of the surface expansion of a solid depends on its coefficientindilationshallow.
Examples of surface thermal expansion
Between the tile boards, used in residential floors and sidewalks, a small free space is left, which is occupied by the grout, a porous material capable of absorbing part of the expansion suffered by the parts ceramics.
It is common to see mechanics heating a nut attached to a bolt in order to remove it, as the heating causes the nut to dilate, facilitating its removal.
volumetric dilation
volumetric dilationit is the expansion of a body's volume by increasing its temperature. The volumetric expansion is calculated from the coefficientindilationvolumetric of the body.
Examples of volumetric thermal expansion
Screws used in aircraft fuselages can be placed at very low temperatures before being threaded. After threading, the increase in temperature of the screw expands its dimensions, making it almost impossible to remove it later.
Thermal expansion coefficient
While some materials must undergo huge temperature variations for their expansion to become noticeable, others need to have their temperature varied by a few degrees so that differences in their dimensions.
The physical property that determines the ease or difficulty of the material having its dimensions changed by a temperature variation is called thermal expansion coefficient.

With the increase in temperature, the molecules of a body start to occupy a larger space.
Lookalso: Calorimetry
Each material has its own coefficient of thermal expansion, which can be of three distinct types: coefficient of dilationlinear, shallow and volumetric. To calculate the expansion suffered by a body, we use only one of these coefficients, determined according to the shape presented by the body.
Despite suffering surface and volumetric dilation, elongated bodies that have linear symmetry, such as cables and wires, are subject to expansion in their length much greater than the expansion in their area or volume.
The expansion coefficients linear, shallow and volumetric are denoted, respectively, by the Greek letters α, β, and γ, and its unit of measure is ºC-1.
The effect of thermal expansion of solids is of great commercial and technological importance. Building construction, for example, uses materials that are often exposed to large and sometimes sharp variations in temperature. In this case, it is essential to know the expansion coefficients of each material used in civil construction in order to avoid the appearance of cracks and other structural defects.
Relationship between expansion coefficients of solids
Bodies with different symmetries made of the same material undergo different forms of expansion. An iron bar, for example, undergoes linear expansion, while a sheet of the same material undergoes surface expansion. This is because the coefficient of surface expansion is twice the coefficient of expansion linear, while the volumetric expansion coefficient is three times greater than the expansion coefficient linear. Watch:
Do not stop now... There's more after the advertising ;)
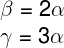
α – linear expansion coefficient
β – surface expansion coefficient
γ – volumetric expansion coefficient
Thermal dilation in bridges
The effects of thermal expansion are especially important in constructions that cannot deform or crack their structure, such as bridges. That's why, in this type of construction, several expansion joints are used.
The image below shows the expansion joint of a bridge. Watch:

Expansion joints reduce the chances of cracking as a result of the expansion of the concrete in the bridges.
Thermal expansion formulas
Check below the formulas used to calculate the linear, superficial and volumetric expansions of solids.
Linear dilation formula
The linear dilation formula can be presented in two ways: one to calculate the final body size and another to calculate the length variation suffered during dilation:

L – final length
L0 – initial length
ΔT - temperature variation
ΔL – length variation
Surface dilation formula
Like the linear expansion formula, the surface expansion formula can also be written in two different ways:

s – final area
s0 – initial area
ΔT - temperature variation
S - area variation
Volumetric expansion formula
Finally, we have the expressions that allow us to calculate the final volume of a body or its volumetric variation:
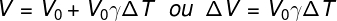
V - Final Volume
V0 – initial volume
ΔT - temperature variation
ΔV – volume variation
Summary
When a solid is heated, its molecules start to vibrate more widely, taking up more space. Depending on the heating and expansion coefficient of the material, the effect can be observed with the naked eye.
The surface and volumetric expansion coefficients of the same homogeneous material (made of a single substance) are, respectively, double and triple the linear expansion coefficient.
Every body undergoes all three types of dilation simultaneously, however, one of them is more significant than the others, as it is more privileged by the shape of the body.
Exercises on thermal expansion
A 2.0 m long iron bar whose coefficient of linear expansion is α=1.2.10-5 °C-1 it is at room temperature (25ºC). This body is then exposed to a heat source, reaching, at the end of its heating, a temperature of 100°C.
Determine:
a) the expansion suffered by the bar.
b) the final length of the bar.
c) the surface and volumetric expansion coefficients of the material from which this bar is made.
Resolution
a) To calculate the expansion suffered by the bar, we need to remember that its shape is linear, so this is the most important form of expansion suffered by it. Using the linear dilation formula, we will have:
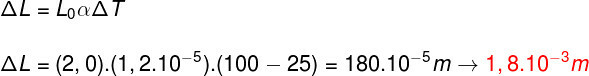
According to the above result, this bar would undergo an expansion of 1.8 mm in its length.
b) The final length of the bar can be easily found, since we already know the expansion suffered by it. Its final length will be 2.0018 m (2 meters and 1.8 mm)
c) The surface and volumetric expansion coefficients are multiples of the linear expansion coefficient. Their values are, respectively, 2,4.10-5 °C-1and 3,6.10-5 °C-1.
By Me. Rafael Helerbrock
Determine the modulus of the coefficient of surface expansion of a 5.0 m long homogeneous steel beam which, when heated to 50 °C, has a linear expansion of 5.10-3 m.
Knowing that a solid and homogeneous material has a constant volumetric expansion coefficient equal to 1.2.10-5 °C-1, determine the coefficient of surface expansion of this material and check the correct alternative:


