The functions of the 2nd degree have several applications in Mathematics and help Physics in various situations in the movement of bodies in the area of Kinematics and Dynamics. Its formation law, where f (x) = ax² + bx + c, describes a parabolic path of concavity facing up (descending - minimum point) or concavity facing downwards (ascending - point maximum). Note the resolution of problem situations below:
Example 1
The movement of a projectile, launched vertically upwards, is described by the equation y = – 40x² + 200x. Where y is the height, in meters, reached by the projectile x seconds after launch. The maximum height reached and the time that this projectile remains in the air correspond, respectively, to:
Resolution:
See the movement graph:

in the expression y = –40x² + 200x the coefficients are a = –40, b = 200 and c = 0.
We will use the expression Yv to obtain the maximum height reached by the object:

The object reached the maximum height of 250 meters.
We will use the expression Xv to obtain the object's rise time:

The projectile took 2.5s to reach maximum height, taking another 2.5s to return to the ground, because in the vertical movement the ascent time is equal to the descent time. Therefore, the projectile remained in the air for 5 s.
Example 2
An object was launched from the top of an 84 m high building with an initial velocity of 32 m/s. How long did it take to reach the ground? Use high school math expression d = 5t² + 32t, which represents the free fall movement of the body.
Resolution:
The body traveled a distance of 84 m which corresponds to the height of the building. Therefore, when substituting d = 84, it is enough to solve the 2nd degree equation formed, determining the value of time t, which will be the root of the equation.
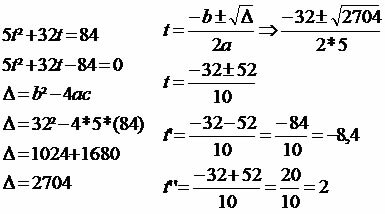
by Mark Noah
Graduated in Mathematics
Brazil School Team
2nd degree function - Roles - Math - Brazil School
Source: Brazil School - https://brasilescola.uol.com.br/matematica/problemas-envolvendo-funcoes-2-grau.htm
