THE clapeyron equation is a mathematical expression that relates quantities such as pressure (P), volume (V), temperature (T) and the number of particles (n) that make up a perfect or ideal gas. This equation is named after the French physicist Paulemileclapeyron and can be understood as a generalization of the empirical laws of the gasesperfectinGay-Lussac, Charles and Boyle. It was fundamental for the study of ideal gases and enabled the evolution of Thermal machines, making them more and more efficient over the last few decades.
See too: Physics Discoveries That Happened By Accident
Clapeyron's Equation: ideal gas law
Clapeyron's equation is derived from three empirical laws, that is, laws that were determined from experiments. Such laws explain the behavior of gases in gas transformationsisovolumetric (Gay-Lussac law), isobaric (Charles law) and isotherms (Boyle's law). According to these laws:
in the transformations isovolumetric, the ratio between pressure and thermodynamic temperature of an ideal gas remains constant;
in the transformations isobaric, the ratio between the volume and thermodynamic temperature of an ideal gas is constant;
- in the transformations isotherms, the product of pressure and volume of an ideal gas remains constant.
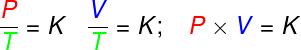
P – pressure (Pa – pascal)
V – gas volume (m³)
T – thermodynamic gas temperature (K – kelvin)
From the three laws above, the Clapeyron equation determines what the value of this constant (K) obtained in each of the aforementioned transformations. According to Clapeyron's equation, this constant is equal to the number of moles multiplied by a constant R, known as the universal constant of ideal gases, and equal to Boltzmann constant multiplied by Avogadro's number.
Formula
Check out the formula that is known as equationinClapeyron:
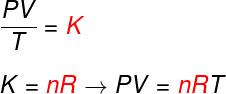
no – number of moles (mol)
R – universal constant of ideal gases (R = 0.082 atm.l/mol. K or 8.314 J.mol/K)
Analyzing the Clapeyron equation, it is possible to see that the pressure exerted by ideal gases is directly proportional to temperature and also to number of moles. Furthermore, pressure is inversely proportional to volume occupied by gas.
O ideal gas model made possible by the Clapeyron equation is widely used for the development of machines powered by fluids, such as steam powered machines and internal combustion engines.
Several important results could be obtained from the Clapeyron equation. One of them, for example, predicts that 1 mole of any ideal gas occupies a volume of 22.4 l when subjected to the pressure of 1 atm (1,01.105 Pa) and at the temperature of 273K (0°C).
See too: Most Important Concepts in Thermology
Solved Exercises on the Clapeyron Equation
Question 1 —(UECE) A gas that can have its temperature, volume and pressure related by PV = nRT has the following characteristics:
a) very large average distance between molecules in order to disregard intermolecular interactions, except when colliding; molecules undergo elastic collisions.
b) very small mean distance between molecules; molecules undergo inelastic collisions.
c) very large mean distance between molecules in order to disregard intermolecular interactions, except when colliding; molecules undergo inelastic collisions.
d) very large mean distance between molecules and with strong intermolecular interactions; molecules undergo elastic collisions.
Resolution:
In the ideal gas model, a large number of dimensionless particles move at high speed in random directions. The only interaction suffered by these particles are perfectly elastic collisions, so the correct alternative is letter a.
Question 2 —(UFRGS) Consider the following statements about ideal gases.
I. The constant R present in the equation of state for gases pV = nRT has the same value for all ideal gases.
II. Equal volumes of different ideal gases, at the same temperature and pressure, contain the same number of molecules.
III. The average kinetic energy of the molecules of an ideal gas is directly proportional to the absolute temperature of the gas.
Which ones are correct?
a) Only I
b) Only II
c) Only III
d) Only I and II
e) I, II and III
Template:
Let's look at the alternatives:
I. correct, hence it is called the universal constant of ideal gases.
II. correct. According to Clapeyron's equation, if two ideal gases have the same pressure, temperature and volume, then the number of particles in those gases is equal.
III. correct. According to the energy equipartition theorem, the kinetic energy of particles in an ideal gas is proportional to its temperature.
Therefore, the correct alternative is the letter e.
Question 3 — (UFJF) Men like Clapeyron, Boyle, Mariotte, Gay-Lussac, van der Walls, among others, have developed important studies involving the properties of gases. The behavior of real gases approximates to ideal gases at low pressure conditions, as well as to gases contained in a large volume and gases held at high temperatures. Consider that, in a laboratory experiment, a container of volume V is completely closed and contains 1 mol of an ideal gas under a pressure of 4.0 atm is subjected to an expansion at a constant temperature equal to 127 º C and that the behavior of this gas is that of an ideal gas, as shown in graphic.
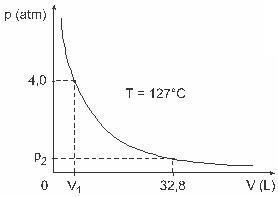
In this case, when the gas is occupying a volume equal to 32.8 L, the pressure exerted by it will be:
(Given: the universal constant of perfect gases is R = 0.082 atm. L/mol. K.)
a) 0.32 atm
b) 0.40 atm
c) 1.0 atm
d) 2.0 atm
e) 2.6 atm
Resolution:
Letter C.
To solve the exercise, we need to apply the data to the Clapeyron equation, but before that we need to transform the temperature, which is in degrees Celsius, to kelvin. Check out:

By Rafael Hellerbock
Physics teacher
Source: Brazil School - https://brasilescola.uol.com.br/fisica/a-equacao-clapeyron.htm
