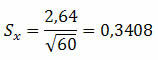Algebraic fraction simplification

Whenever the word “algebraic” is used for a numeric expression, it means that that expression has at least one unknown, that is, a letter or symbol used to represent a number unknown. Thus, a algebraic fraction, in turn, is nothing more than a fraction that has at least one unknown in the denominator (bottom of fraction). Therefore, the simplification of algebraic fractions follows the same foundation as the simplification of numerical fractions.

Examples of algebraic fractions are:

1)

2x
4y

2)

4y² – 9x²
2y + 3x

Simplifying algebraic fractions

Simplifying an algebraic fraction follows the same foundation as simplifying a numerical fraction. It is necessary to divide numerator and denominator by the same number. Note an example of fraction simplification:

 30  =  15  =  5 =  1
 60 30 10 2 

The fraction above was simplified by 2, then by 3 and then by 5. To support the procedure of simplification of algebraic fractions, we will rewrite the first fraction above in its factored form:

30 =  2·3·5
60 2·2·3·5

Note that the numbers 2, 3, and 5 are repeated in the numerator and denominator and that they were exactly the same numbers that the fraction was simplified by. In the context of algebraic fractions, the procedure is similar, as it is necessary to factor the polynomials present in the numerator and denominator. After that, we must assess whether it is possible to simplify some of them.

Examples

1) Simplify the following algebraic fraction:

4x²y³
16xy⁶

Factor each of the unknowns and numbers present in the fraction:

4x²y³
16xy⁶

2·2·x·x·y·y·y
2·2·2·2·x·y·y·y·y·y·y

Now perform as many divisions as possible, as you did earlier for the numeric fraction: The numbers that appear in both the numerator and denominator disappear, that is, they are "cut". It is also possible to write that the result of each of these simplifications is 1. Watch:

2·2·x·x·y·y·y
2·2·2·2·x·y·y·y·y·y·y

x
2·2·y·y·y

x
4y³

2) Simplify the following algebraic fraction:

4y² – 9x²
2y + 3x

Note that the numerator of this algebraic fraction falls into one of the cases of notable products, that is, the two square difference. To factor it in, just rewrite it in its factored form. After that, it is possible to “cut” the terms that appear in both the denominator and the numerator as in the previous example. Watch:

4y² – 9x²
2y + 3x

= (2y + 3x) (2y - 3x)
2y + 3x

= 1·(2y – 3x)

= 2y + 3x

3) Simplify the following algebraic fraction:

The²(y² – 16x²)
ay + 4ax

As previously done, factor the polynomials present in the numerator and denominator. After that, carry out the divisions that are possible.

The²(y² – 16x²)
ay + 4ax

= The·The·(y + 4x)(y - 4x)
a·(y + 4x)

Note that the numerator has been factored using the two square difference and the denominator was factored through the common factor. In addition, the term a² can be written as the product a·a. Finally, perform as many divisions as possible. Namely, a by a and (y + 4x) by (y + 4x):

Do not stop now... There's more after the advertising ;)

The·The·(y + 4x)(y - 4x)
a·(y + 4x)

= 1·1·(y – 4x)

= y - 4x

Factorization cases are of paramount importance to simplify algebraic fractions. Below are listed the most important cases and some pages where they can be found in more detail.

Factoring of algebraic expressions

A polynomial can be written in its factored form if it can be expressed in one of the four forms below. The results presented are their factored form or examples of how to factor them:

1 - Common factor

If all the terms of the polynomial have an unknown or some common number, it is possible to put them in evidence. For example, in the 4x polynomial² + 2x we can put 2x in evidence. The result will be:

4x² + 2x = 2x (2x + 1)

Note that when performing the multiplication indicated on the second member (right side of the equality), the result will be precisely the first member (left side of equality), due to the distributive property of the multiplication.

2 – Grouping

In view of the previous case, a polynomial that has four terms can be factored by grouping, joining the common terms two by two, and later be factored again if the results leave this possibility. The 2x + bx + 2y + by polynomial, for example, can be factored as follows:

2x + bx + 2y + by

x (2 + b) + y (2 + b)

Note that (2 + b) repeats in both new terms. So, we can put it in evidence:

x (2 + b) + y (2 + b)

(2 + b)(x + y)

3 – Perfect square trinomial

Whenever a polynomial is a perfect square trinomial, it is written equivalent to one of the following three expressions arranged on the left and in red.

x² + 2x + a² = (x + a)(x + a)

x² – 2x + a² = (x - a)(x - a)

x² - a² = (x + a)(x - a)

The right side is the factored shape of the polynomial, which can be used for the algebraic fraction simplification.

4 – Sum or difference of two cubes

Whenever the polynomial is in the next shape or can be written to it, it will be a sum of two cubes.

x³ + 3x²at + 3x² + the³ = (x + a)³

x³ – 3x²at + 3x² - a³ = (x - a)³

Again, the left-hand side, in red, is the polynomial that can be factored and rewritten like the expressions on the right-hand side.

By Luiz Paulo Moreira
Graduated in Mathematics

Would you like to reference this text in a school or academic work? Look:

SILVA, Luiz Paulo Moreira. "Algebraic fraction simplification"; Brazil School. Available in: https://brasilescola.uol.com.br/matematica/simplificacao-fracao-algebrica.htm. Accessed on June 28, 2021.

Trinomial of the type x² + Sx + P

Factorization, Algebraic expression, Expression, Algebraic expression factorization, Sum, product, Middle terms, Extreme terms, Trinomial, Trinomial of type x2 + Sx + P.