A triangle is classified as scalene when all its sides have different measurements. When comparing the sides of the triangle, it can be isosceles, when it has two congruent sides, equilateral, when it has all congruent sides, and scalene, when it has all sides with different measurements.
The scalene triangle is the most common of the triangles day to day. To calculate its area, we can use the most common formula, which is the product of the base and the height divided by two, yet, when we only know the measurement of its sides, you can use Heron's formula. The perimeter of the scalene triangle is the sum of all its sides.
Read too: What are the classification criteria for triangles?
scalene triangle
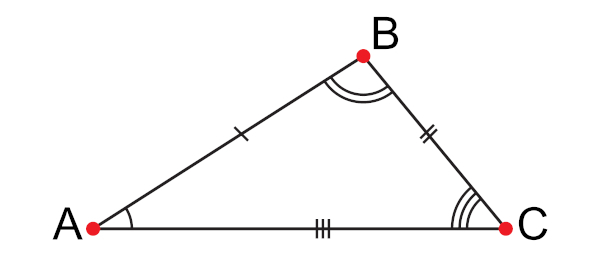
The triangle is the polygon most studied in plane geometry. In the midst of studies in this area, some classifications for this figure emerge, and one of them is its classification as a scalene triangle.
A triangle is classified as a scalene when its sides are of different lengths. |
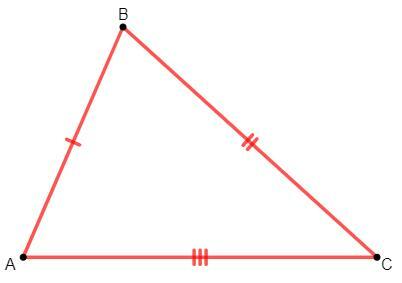
The sides are AB, AC and BC. Since the triangle is scalene, we have AB ≠ AC ≠ BC.
Scalene triangle angles
As a result of the sides always having different measures, in a scalene triangle, theangles also têin your measurements always distinct.

As in every triangle, the sum of the internal angles equals 180°. In the scalene triangle, this is no different, that is, α + ꞵ + γ = 180º.
Perimeter of the scalene triangle
To calculate the perimeter of a scalene triangle, as well as any other triangle, we perform thesum on your three sides.

P = a + b + c
Example:
Calculate the perimeter of the triangle:
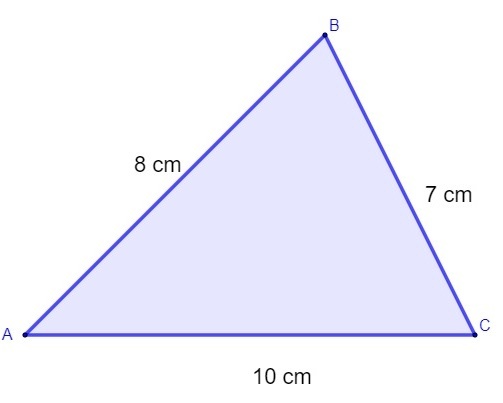
P = 8 + 7 + 10
P = 15 + 10
P = 25 cm
See too: What are the remarkable points of a triangle?
Scalene Triangle Area
To calculate the area of any triangle, just calculate the product between the base length and O tall and share for two:
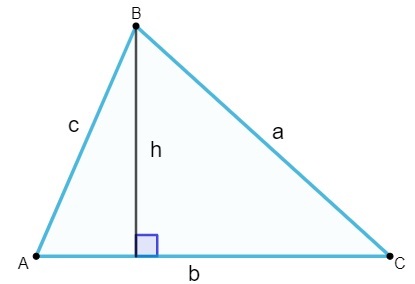

Example:
Calculate the area of a triangle that has a base measuring 30 cm and a height measuring 22 cm.

Heron's formula
We can calculate the area of the scalene triangle also byHeron's formula. When we don't know the height of a triangle, Heron's formula allows us to calculate the area of that polygon, as long as the length of its three sides is known. Using the triangle with sides a, b, c, to find the area of the triangle by Heron's formula, we have to calculate the semiperimeter P, which is half the perimeter of the triangle, that is:

Knowing the semiperimeter, the area of a triangle using the Heron formula is calculated by:
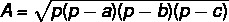
Example:
Calculate the area of a scalene triangle that has sides measuring 14 cm, 9 cm, and 7 cm.
As we do not know your height, therefore, it is convenient to use Heron's formula to find your area.
First we will calculate the semiperimeter P:

Now that we know the semiperimeter, let's calculate the area of this triangle:

See too: Rectangle triangle - triangle that has one of its angles measuring 90º
solved exercises
Question 1 - On a farm, a region was set aside for the planting of corn. When performing the measurements, it was possible to see that this region was limited by a scalene triangle, as shown in the following image:
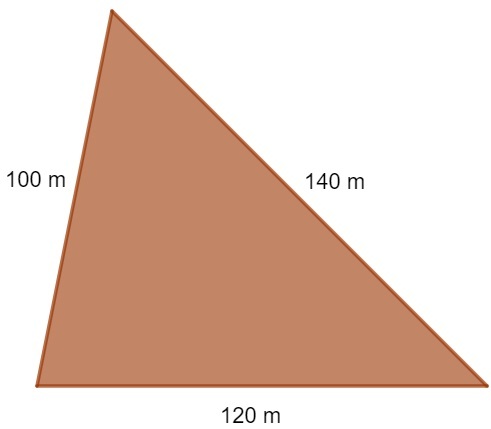
For the safety of the crop, the farmer decided to fence off this area with barbed wire whose meter costs R$ 0.80. Knowing that the fence will have 4 strands of barbed wire around the perimeter, the minimum amount spent on barbed wire to meet these requirements will be:
A) BRL 288
B) BRL 576
C) BRL 934
D) BRL 1152
E) BRL 1440
Resolution
Alternative D
First we will calculate the perimeter of the lot.
P = 120 + 100 + 140 = 360 m
Knowing that he will do 4 laps over this terrain, we have to:
4P = 360 · 4
4P = 1440 m
Finally, as each meter costs R$ 0.80, we have to:
1440 · 0,80 = 1152
Question 2 - At the request of an architect, a woodworker will make a wooden scalene triangle. The measurements for the sides of the figure given by the architect were: 2.5 meters, 3.5 meters and 5 meters. Based on these measurements, the area of this triangle, in square meters, is:
A) greater than 3.0 m² and less than 3.5 m².
B) larger than 3.5 m² and smaller than 3.9 m².
C) greater than 4.0 m² and less than 4.5 m².
D) greater than 4.6 m² and less than 4.9 m².
E) greater than 5.0 and less than 5.5 m².
Resolution
Alternative C
Since we don't know the height, let's use Heron's formula to find the table area. First we will calculate your semiperimeter:
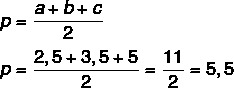
Now let's calculate the area:
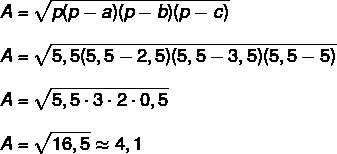
We then know that 4.1 m² is between 4.0 and 4.5.
By Raul Rodrigues de Oliveira
Maths teacher
Source: Brazil School - https://brasilescola.uol.com.br/matematica/triangulo-escaleno.htm
