One straight it is a set of points. Its geometric representation is given by a flat geometric figure, formed by a lineonly, straight, infinite for two directions and, therefore, does not make any curve in its entirety.
Two straight contained in the same flat they can interact in some different ways, generating concepts, definitions and properties. The set of possible interactions between two lines is called relative positions. Are they:
parallel lines
two straights are parallel when they do not have any common ground along their entire length. An interesting property about these straight is that the distance between them will always be the same, regardless of the point chosen to measure them. The following image is an example of two parallel lines:
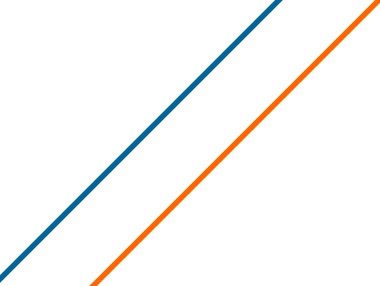
Also read: What are parallel lines
Competing lines
two straights are competitors when they have a single point of intersection. Competing lines form four angles, congruent two to two. When one of them measures 90°, the concurrent lines are called perpendicular. The image shows an example of competing lines:
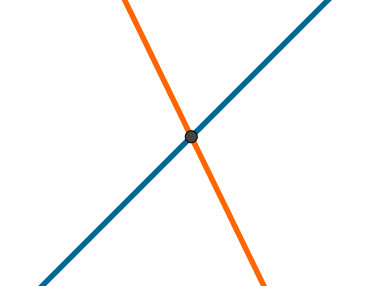
Also read: What are straight competitors
when two straight they are competitors, the angles formed can be classified as adjacent or opposite by the vertex. Two angles opposite by the vertex are congruent. Two adjacent angles are supplementary. Furthermore, two perpendicular lines are always concurrent, but not always two concurrent lines are perpendicular.
Also read: Types of lines
Coincident lines
Two lines are coincident when all points on the first are also points on the second and vice versa.
It is common to find authors who state: two lines are coincident when they have two or more points in common. This type of relationship is based on a result of geometry: if two lines have at least two points in common, then all points on the first are points on the second.
We can also say that two straightcoincident are actually a single line, as shown in the following figure:

By Luiz Paulo Moreira
Graduated in Mathematics
Source: Brazil School - https://brasilescola.uol.com.br/matematica/posicoes-relativas-entre-duas-retas.htm

