Practice your knowledge about atmospheric pressure with the exercises and resolve your doubts with the commented solutions.
Question 1
Atmosphere is a layer of gases that surrounds a celestial body. Regarding the concept of atmosphere, the following statements are correct:
Atmospheric pressure at sea level is generally greater than atmospheric pressure at higher altitudes.
Atmospheric pressure varies with temperature, with pressure increasing as temperature decreases.
Atmospheric pressure is higher in regions close to the Equator due to the greater air density in this region.
a) Only 1
b) Only 2
c) Only 1 and 2
d) Only 2 and 3
e) All
Answer: c) Only 1 and 2.
Atmospheric pressure is inversely related to altitude and temperature. Furthermore, it is not directly linked to the equator.
Question 2
A mercury barometer positioned at sea level records a pressure of 760 mmHg. With changes in atmospheric climate such as air temperature and humidity, the same barometer increases 15 mmHg in value registered. Right now. What will be the atmospheric pressure in atm?
Answer: Approximately 1.019 atm.
We just need to solve a rule of three.
Question 3
In a suction pump, air is removed from a metallic cylinder until its internal pressure registers Pa. Determine the pressure difference in atmospheres between the outside, at
, and the inside of the cylinder.
Answer: Approximately 0.79 atm.
With atmospheric pressure being 101,000 Pa and internal pressure being 80,000 Pa, the pressure difference will be:
101,000 - 80,000 = 21,000 Pa.
As 1 atm = 101,000 Pa, to know how much 21,000 Pa is equivalent to in atm, just use a rule of three.
Question 4
(CBM-AP 2012) A group of firefighters are undergoing diving training in a lake. The instructor informs you that the atmospheric pressure at the surface of the lake is approximately 101 kPa. In He then asks the group of soldiers at what depth the pressure is twice atmospheric pressure. Considering that the specific mass of water (ρ) is equal to and that the acceleration of gravity (g) is equal to
, select the alternative that presents the correct answer to be reported to the instructor.
a) 5.05 m
b) 10.1 m
c) 20.2 m
d) 50.5 m
e) 101 m
For the pressure to be twice that of atmospheric pressure, it must be:
At the surface of the fluid there is already an action of 101 kPa by the atmosphere. Therefore, the pressure in the liquid must also be 101 Kpa.
Question 5
(Enem 2013) To carry out an experiment with a PET bottle filled with water, the side of the bottle was punctured in three positions at different heights. With the bottle covered, the water did not leak through any of the holes, and, with the bottle uncovered, water flow was observed as shown in the figure.
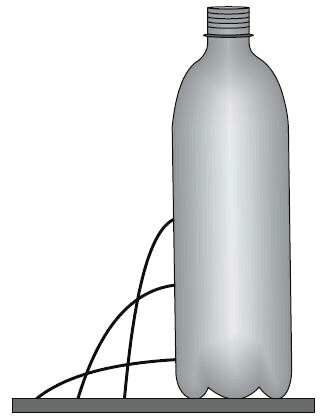
How does atmospheric pressure interfere with water flow, in situations with the bottle capped and uncovered, respectively?
a) Prevents water from escaping, as it is greater than the internal pressure; does not change the flow speed, which only depends on the pressure of the water column.
b) prevents water from escaping, as it is greater than the internal pressure; changes the flow velocity, which is proportional to the atmospheric pressure at the height of the hole.
c) Prevents air from entering, as it is lower than the internal pressure; changes the flow velocity, which is proportional to the atmospheric pressure at the height of the hole.
d) Prevents water from escaping, as it is greater than the internal pressure; regulates the flow speed, which only depends on atmospheric pressure.
e) Prevents air from entering, as it is lower than the internal pressure; does not change the flow speed, which only depends on the pressure of the water column.
With the bottle capped, the hydrostatic pressure inside the bottle is equal to the atmospheric pressure outside, so, due to equilibrium, there is no flow.
With the bottle uncapped, in addition to the hydrostatic pressure inside the bottle, there is also atmospheric pressure, with the internal pressure being greater than the external pressure.
Question 6
(Fuvest 2019) Large commercial planes fly at altitudes where the air is thin and atmospheric pressure is low. Due to this, their interior is pressurized at a pressure equal to atmospheric pressure at an altitude of 2,000 m. The figure shows the graph of atmospheric pressure as a function of altitude.
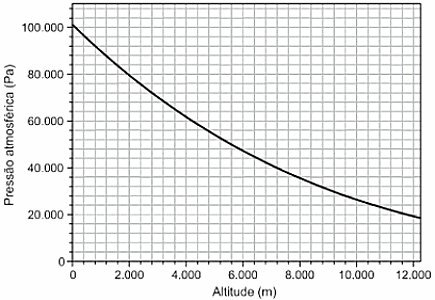
The force, in N, to which a flat glass window, measuring 20 x 30 cm², is subjected, in the passenger cabin at an altitude of 10,000 m, is approximately
a) 12,400
b) 6,400
c) 4,800
d) 3,200
e) 1,600
Objective: calculate the force in Newtons acting on the window.
Inside the plane.
Pressure is force over area. This way we can determine the force.
In the plane, pressure is equal to atmospheric pressure at an altitude of 2000 m. From the graph we obtain 80,000 Pa.
The area of the window, in square meters, is:
Therefore, the force inside is:
Outside the plane.
The pressure is equal to atmospheric pressure at an altitude of 10,000 m. From the graph we obtain about 26,000 Pa.
The resultant force vector that acts on the window will be:
4800 - 1560 = 3 240 N
Learn more about atmospheric pressure.
See too:
- What is Atmosphere?
- Air Properties
- Hydrostatics: density, pressure, thrust and formulas
- Layers of the Earth's atmosphere and their characteristics
- Stevin's Theorem: Fundamental Law of Hydrostatics
ASTH, Rafael. Atmospheric pressure exercises solved.All Matter, [n.d.]. Available in: https://www.todamateria.com.br/exercicios-de-pressao-atmosferica/. Access at:
See too
- Atmospheric pressure
- Hydrostatic exercises
- Hydrostatic Pressure
- Thermometric Scales - Exercises
- Kinematics: Exercises commented and solved
- Exercises on thermodynamics

