Fractions have two types of representation, one geometric (drawing) and the other in the form of mathematical expression. It is important to remember that a fraction is a representation of a part of a whole.
To have a fractional representation we must first constitute the whole integer.
The following figure represents an integer. We can divide the pizza into several parts.

The pizza has been divided into eight equal parts, each part will represent a fraction according to the integer. If we remove a piece, it will correspond to one eighth of the integer.

Every fraction in the form of a mathematical expression is represented according to a general rule, its terms are given names: numerator and denominator. The numerator is intended to represent a certain part of the integer. The denominator represents how many parts the integer has been divided. The numerator and denominator are separated by a slash, which is also intended to express the division operation.
We can represent the parts of the divided pizza as follows:
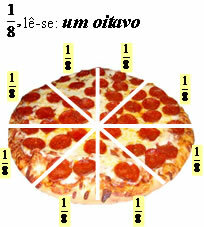
Knowing that a fraction must be represented by a numerator and a denominator, it is easy to understand its nomenclature. The reading of a fraction will depend on its denominator.
The nomenclature of a fraction can be divided into two groups:
- the first comprises denominators equal to 2, 3, 4, 5, 6, 7, 8, 9, 10, 100, 1000.
- the second comprises denominators that do not belong to the first group, such as 12, 20, 51.
For denominators equal to 2, 3, 4, 5, 6, 7, 8, 9, 10, 100, 1000, the reading of fractions is as follows:

Second group: considering that the denominator is any other number, we add the word “avos” in its reading. 
by Mark Noah
Graduated in Mathematics
Brazil School Team
Fraction - Math - Brazil School
Source: Brazil School - https://brasilescola.uol.com.br/matematica/nomenclatura-fracao.htm
