A symmetry is anything that can be divided into parts, so that the parts coincide perfectly when superimposed. This is an important concept studied in geometry. We can see the presence of symmetry in art, geometry, biology and other areas of knowledge.
There are different types of symmetry: reflective, translational, and rotational. Symmetry and asymmetry are opposite concepts, that is, either a figure is symmetrical or asymmetrical. To check whether a figure is symmetrical or asymmetrical, we draw a straight line, dividing it. If it is formed in two ways that coincide perfectly when superimposed, then this figure is symmetrical, and the line is known as the axis of symmetry; otherwise, the figure will be asymmetrical.
Read too: Flatness of geometric solids
Topics of this article
- 1 - Summary on symmetry
- 2 - What is symmetry?
-
3 - What are the types of symmetry?
- reflective symmetry
- translational symmetry
- rotation symmetry
- 4 - Difference between symmetry and asymmetry
- 5 - Importance of symmetry
Summary about symmetry
- A shape is known as symmetrical if, when divided into parts, these parts coincide perfectly when superimposed.
- A figure can be symmetrical or asymmetrical.
- The symmetric figure can translate or rotate without changing the figure.
- The asymmetric figure is opposite, its rotation or translation changes the figure.
- There are three types of symmetry, they are:
- reflective symmetry: when the form can be divided into two equal parts.
- Translation symmetry: when a figure is moved without rotating, in any direction.
- Rotational symmetry: when a figure is rotated relative to one of its points.
Do not stop now... There's more after the publicity ;)
What is symmetry?
The symmetry is one of the first concepts studied in geometry. It is linked to the harmony of form, to beauty; symmetry is all that we can divide into parts, so that the parts coincide perfectly when overlapping, which means that when we divide this shape, we will find two shapes identical.

We can see the presence of symmetry in geometry, art, architecture, nature, among other places in our daily lives. O The axis of symmetry of a figure is a line passing through the center of the figure, dividing it into symmetrical parts.
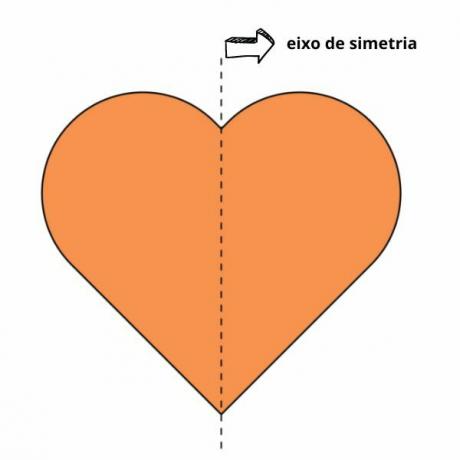
What are the types of symmetry?
There are three types of symmetry, reflective, translational and rotational.
reflective symmetry
As the name suggests, it is linked to the reflex; is when one image is the reflection of another.
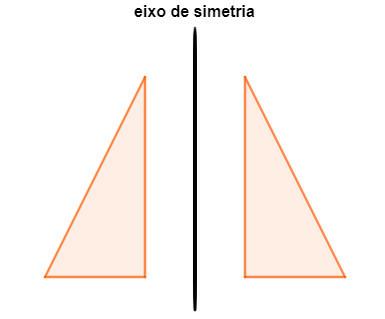
It is important to realize that, on reflection, the triangle it changes the opposition of the sides, because in this case it is as if the first triangle were being reflected in a mirror by the second triangle.
We can verify this symmetry in nature, in landscapes with water for example:

Reflection symmetry can also be known as mirror symmetry or axial symmetry, in which case it is as if the axis does the same thing as the mirror.
Symmetry of translation
We know it as translation when there is a displacement of the figure. In this case, the figure will only move forwards, backwards, sideways, so it cannot rotate.

It is important to point out that, in translation, the figures have same area, therefore, there cannot be an increase in area, changes in its shape, or even a rotation, since the rotation of the figure is another case of symmetry.
Symmetry of rotation
It is the geometric transformation in which the figure is obtained after rotating the main figure. Rotation can be done clockwise or counterclockwise.

Difference between symmetry and asymmetry
As we have seen, symmetry is when we have two figures that overlap perfectly; an asymmetry is the opposite case, that is, when there is no pattern or similarity between the parts of the figure. So we can say that the concepts of symmetry and asymmetry are contrary, either we have symmetry or we have asymmetry. Each of the cases has an important function in the study of geometry.
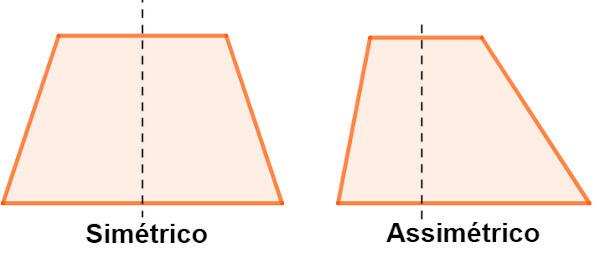
importance of symmetry
The study of symmetry is present in several areas of knowledge, such as biology, more specifically in the study of body symmetry in living beings and in nature. This is an important area of biology study, as it is based on it that some zoological classifications arise.

We can also notice the importance of symmetry in art and architecture. Symmetry is linked to beauty and harmony, which is why it is present in various works of art and buildings.
By Raul Rodrigues de Oliveira
Maths teacher
Congruence cases of triangles.
Learn how to build the trigonometric circle, in addition to understanding how the reduction to the first quadrant works and how to study trigonometry through it.
Click here and learn what a symmetric matrix is. Know its properties and discover how it differs from an antisymmetric matrix.
Find out what the parable is, what its elements and properties are. Know also its applications in other areas of knowledge.
