A proportion golden or divine proportion is an equality associated with ideas of harmony, beauty and perfection. Euclid of Alexandria, Greek mathematician who lived around 300 BC. C., was one of the first thinkers to formalize this concept that until today intrigues researchers from different areas.
The reason for this interest is that the golden ratio can be observed in an approximate way in nature, including in the seeds and leaves of plants and in the human body. Consequently, the golden ratio is the subject of study by different professionals, such as biologists, architects, artists and designers.
Read too: Number pi — one of the most important constants in mathematics
Topics of this article
- 1 - Summary of the golden ratio
- 2 - How to calculate the golden number?
- 3 - Golden ratio and the Fibonacci sequence
- 4 - Golden ratio and the golden rectangle
-
5 - Applications of the golden ratio
- Golden Ratio in Architecture
- Golden ratio in the human body
- golden ratio in art
- Golden ratio in nature
- Golden Ratio in Design
- 6 - Solved exercises on golden ratio
Summary about golden ratio
The golden ratio is the ratio for \(a>b>0\) such that
\(\frac{a+b}a =\frac{a}b\)
Under these conditions, the reason TheB is called the golden ratio.
The golden ratio is connected to conceptions of balance, purity and perfection.
The Greek letter ϕ (read: fi) represents the golden number, which is the constant obtained from the golden ratio.
In the Fibonacci sequence, the quotients between each term and its predecessor approach the golden number.
The golden rectangle is a rectangle whose sides are in the golden ratio.
What is golden ratio?
Consider a line segment divided into two pieces: the larger one of measure The and the smallest B. realize that a+b is the measure of the entire segment.

the golden ratio is equality among the reasons\(\mathbf{\frac{a+b}a}\) It is \(\mathbf{\frac{a}{b}}\), i.e
\(\frac{a+b}a =\frac{a}b\)
In this context, we say that The It is B are in golden ratio.
But for what values of The It is B do we have the golden ratio? That's what we'll see next.
Do not stop now... There's more after the publicity ;)
How to calculate the golden number?
The reason \(\frac{a}b\)(or, likewise, the reason \(\frac{a+b}a\)) results in a constant called the golden number and represented by the Greek letter ϕ. Thus, it is common to write
\(\frac{a+b}a =\frac{a}b=ϕ\)
To calculate the golden number, let's consider the golden ratio for b = 1. Thus, we can easily find the value of The and get ϕ from equality \(\mathbf{\frac{a}{b}=ϕ}\).
Note that we can write the golden ratio as follows, using the cross multiplication property:
\(a^2=b⋅(a+b)\)
Substituting b = 1, we have
\(a^2=1⋅(a+1)\)
\(a^2-a-1=0\)
Applying Bhaskara's formula for this quadratic equation, we conclude that the positive solution of The é
\(a=\frac{1+\sqrt5}2\)
As The is a measure of a segment, we will disregard the negative solution.
So how \(\frac{a}b=ϕ\), The exact value of the golden number is:
\(ϕ=\frac{1+\sqrt5}2\)
Calculating the quotient, we get The approximate value of the golden number:
\(ϕ≈1,618033989\)
See too: How to solve math operations with fractions?
Golden Ratio and the Fibonacci Sequence
A Fibonacci sequence is a list of numbers where each term, starting from the third, is equal to the sum of the two predecessors. Let's look at the first ten terms of this sequence:
\(a_1=1\)
\(a_2=1\)
\(a_3=1+1=2\)
\(a_4=1+2=3\)
\(a_5=2+3=5\)
\(a_6=3+5=8\)
\(a_7=5+8=13\)
\(a_8=8+13=21\)
\(a_9=13+21=34\)
\(a_{10}=21+34=55\)
As we calculate the quotient between each term and its predecessor in the Fibonacci sequence, we are approaching the golden number ϕ:
\(\frac{a_2}{a_1}=\frac{1}1=1\)
\(\frac{a_3}{a_2}=\frac{2}1=2\)
\(\frac{a_4}{a_3}=\frac{3}2=1.5\)
\(\frac{a_5}{a_4}=\frac{5}3=1.6666…\)
\(\frac{a_6}{a_5}=\frac{8}5=1.6\)
\(\frac{a_7}{a_6}=\frac{13}8=1.625\)
\(\frac{a_8}{a_7}=\frac{21}{13}=1.6153…\)
\(\frac{a_9}{a_8}=\frac{34}{21}=1.61904…\)
\(\frac{a_10}{a_9}=\frac{55}{34}=1.61764…\)
Golden ratio and the golden rectangle
One rectangle where the longest side The and the smaller side B are in golden ratio it's called the golden rectangle. An example of a golden rectangle is a rectangle whose sides measure 1 cm and \(\frac{1+\sqrt5}2\) cm.
Know more: What are directly proportional quantities?
Applications of the Golden Ratio
Note that, until now, we have studied the golden ratio only in abstract mathematical contexts. Next, we will see some applied examples, but care is needed: the golden ratio is not presented exactly in any of these cases. What exists are analyzes of different contexts in which the golden number appears soapproximate.
Golden Ratio in Architecture
Some studies claim that estimates of the number of gold are observed in certain ratios of the dimensions of the Pyramid of Cheops, in Egypt, and the UN headquarters building, in New York.

Golden ratio in the human body
Human body measurements vary from one person to another, and there is no perfect body type. However, at least since Ancient Greece, there have been debates about a mathematically ideal body (and totally unattainable in reality), with measurements related to the golden ratio. In this theoretical context, for example, the ratio of a person's height to the distance between their navel and the ground would be the golden number.
golden ratio in art
There is research on the works “The Vitruvian Man” and “Mona Lisa”, by the Italian Leonardo da Vinci, which suggest the use of golden rectangles.

Golden ratio in nature
There are studies that point to a relationship between the golden ratio and the way in which the leaves of certain plants are distributed on a stem. This arrangement of leaves is called phyllotaxy.

Golden Ratio in Design
The golden ratio is also studied and used in the area of Design as a project composition tool.

Solved exercises on golden ratio
question 1
(Enem) A line segment is divided into two parts in the golden ratio when the whole is to one of the parts in the same ratio that this part is to the other. This proportionality constant is commonly represented by the Greek letter ϕ, and its value is given by the positive solution of the equation ϕ2 = ϕ+1.
Just like the power \(ϕ^2\), the higher powers of ϕ can be expressed in the form \(aϕ+b\), where a and b are positive integers, as shown in the table.
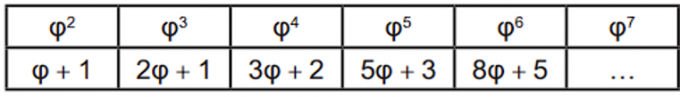
the potency \(ϕ^7\), written in the form aϕ+b (a and b are positive integers), is
a) 5ϕ+3
b) 7ϕ+2
c) 9ϕ+6
d) 11ϕ+7
e) 13ϕ+8
Resolution
As \(ϕ^7=ϕ⋅ϕ^6\), We have to
\(ϕ^7=ϕ⋅ϕ^6 = ϕ⋅(8ϕ+5)\)
Applying the distributive,
\(ϕ^7=8ϕ^2+5ϕ\)
As \(ϕ^2=ϕ+1\),
\(ϕ^7=8⋅(ϕ+1)+5ϕ\)
\(ϕ^7=13ϕ+8\)
E alternative.
question 2
Rate each statement below about the golden number as T (True) or F (False).
i. The golden number ϕ is irrational.
II. The quotients between each term and its predecessor in the Fibonacci sequence approach the value of ϕ.
III. 1.618 is the rounding to three decimal places of the golden number ϕ.
The correct sequence, from top to bottom, is
a) V-V-V
b) F-V-F
c) V-F-V
d) F-F-F
e) F-V-V
Resolution
i. True.
II. True.
III. True.
Alternative A.
Sources
FRANCISCO, S. V. from L. Between the fascination and the reality of the golden ratio. Dissertation (Professional Master's Degree in Mathematics in National Network) – Institute of Biosciences, Letters and Exact Sciences, Universidade Estadual Paulista Júlio de Mesquita Filho. São Paulo, 2017. Available in: http://hdl.handle.net/11449/148903.
SALES, J. from S. The golden ratio present in nature. Completion of course work (Degree in Mathematics), Federal Institute of Education, Science and Technology of Piauí. Piauí, 2022. Available in http://bia.ifpi.edu.br: 8080/jspui/handle/123456789/1551.
By Maria Luiza Alves Rizzo
Math teacher
Understand what it is and how to calculate average speed and population density.
Learn what it is and how to use Bhaskara's formula to solve quadratic equations!
Understand what directly proportional quantities are and learn how to solve problem situations involving this type of relationship.
Learn here how to determine if two quantities or numbers are inversely proportional. Check out examples and exercise on the topic!
Learn here what a proportion is and how to calculate it. See also its main properties and understand what proportional quantities are.
See here the different ways to represent a ratio, see also the definition and some applications of proportion. Learn how to apply these concepts.
Learn to use the compound rule of three to find unknown values and problems with three or four quantities.
Know the rule of three. Understand what direct and inversely proportional quantities are. Know the difference between the simple rule of three and the compound rule.
Numerical Sequences: Fibonacci Sequence.