Due to its shape and some interesting properties, the right triangle was decisive for the origin of trigonometry. In it, we can determine the ascent rate by creating relationships with terms from trigonometry such as sine, cosine and tangent. In the triangle, we have that the sum of the internal angles corresponds to 180º. Knowing that one of the right triangle's angles measures 90º, we determine that the others have measures smaller than 90º, that is, acute and complementary angles. Treble, because they have measures smaller than 90º and complementary, because the sum is equal to 90º.
These acute angles were related to sine, cosine and tangent values according to trigonometric studies. Let's determine in the right triangle, in relation to one of the acute angles, the idea of the rate of rise. Look:
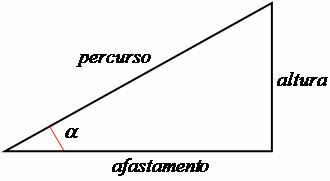
According to the triangle and the elements provided, we can establish three situations in relation to the acute angle α. Look:

The height measurement corresponds to the opposite side of angle α.
The measure represented by the offset corresponds to the adjacent side of angle α.
The path concerns the measurement of the hypotenuse of the right triangle.
According to these relationships, we establish the following trigonometric relationships:

by Mark Noah
Graduated in Mathematics
Brazil School Team
Trigonometry - Math - Brazil School
Source: Brazil School - https://brasilescola.uol.com.br/matematica/propriedades-triangulo-retangulo.htm
