Resistor Association: in series, in parallel and mixed with exercises

Resistor Association is a circuit that has two or more resistors. There are three types of association: parallel, series and mixed.

By analyzing a circuit, we can find the value of the equivalent resistor, that is, the value of the resistance that alone could replace all the others without changing the values ​​of the other quantities associated with the circuit.

To calculate the voltage that the terminals of each resistor is subjected to, we apply the First Ohm's Law:

U = R. i

Where,

U: electrical potential difference (ddp), measured in Volts (V)
R: resistance, measured in Ohm (Ω)
i: intensity of the electric current, measured in Amperes (A).

Association of Series Resistors

When associating resistors in series, the resistors are connected in sequence. This causes electrical current to be maintained throughout the circuit, while electrical voltage varies.

Thus, the equivalent resistance (R_eq) of a circuit corresponds to the sum of the resistances of each resistor present in the circuit:

R_eq = R₁ + R₂ + R₃ +...+ R_no

Association of Parallel Resistors

In the association of resistors in parallel, all resistors are subject to the same potential difference. The electric current being divided by the branches of the circuit.

Thus, the inverse of the equivalent resistance of a circuit is equal to the sum of the inverses of the resistances of each resistor in the circuit:

When, in a parallel circuit, the value of the resistances are equal, we can find the value of equivalent resistance by dividing the value of a resistance by the number of resistances in the circuit, or be:

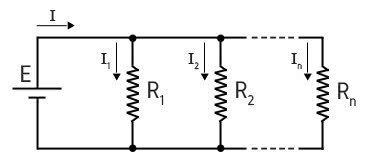
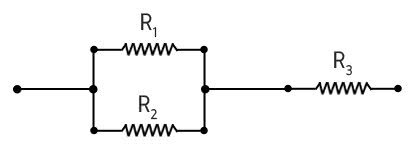
Mixed Resistor Association

In mixed resistor association, the resistors are connected in series and in parallel. To calculate it, we first find the value corresponding to the parallel association and then add the resistors in series.

read

• Resistors
• Electrical Resistance
• Physics Formulas
• Kirchhoff's Laws

Solved Exercises

1) UFRGS - 2018

A voltage source whose electromotive force is 15 V has an internal resistance of 5 Ω. The source is connected in series with an incandescent lamp and a resistor. Measurements are carried out and it is verified that the electric current that passes through the resistor is 0.20 A, and that the potential difference in the lamp is 4 V.

In this circumstance, the electrical resistances of the lamp and resistor are, respectively,

a) 0.8 Ω and 50 Ω.
b) 20 Ω and 50 Ω.
c) 0.8 Ω and 55 Ω.
d) 20 Ω and 55 Ω.
e) 20 Ω and 70 Ω.

See Answer

As the circuit's resistors are connected in series, the current that runs through each of its sections is equal. In this way, the current through the lamp is also equal to 0.20 A.

We can then apply the 1st Ohm's law to calculate the resistance value of the lamp:

U_L = R_L. i

Now, let's calculate the resistor resistance. As we do not know the ddp value between its terminals, we will use the total ddp value of the circuit.

For this, we will apply the formula considering the equivalent resistance of the circuit, which in this case is equal to the sum of all resistances in the circuit. So we have:

U_total = R_eq.i

Alternative: b) 20 Ω and 50 Ω

2) PUC/RJ - 2018

A circuit has 3 identical resistors, two of them placed in parallel with each other, and connected in series with the third resistor and with a 12V source. The current flowing through the source is 5.0 mA.

What is the resistance of each resistor, in kΩ?

a) 0.60
b) 0.80
c) 1.2
d) 1.6
e) 2.4

See Answer

Knowing the value of the total ddp and the current that passes through the circuit, we can find the equivalent resistance:

U_Total = R_eq.i

As the resistances have the same value, the equivalent resistance can be found by doing:

Alternative: d) 1.6

3) PUC/SP - 2018

Determine, in ohm, the resistance value of the equivalent resistor of the association below:

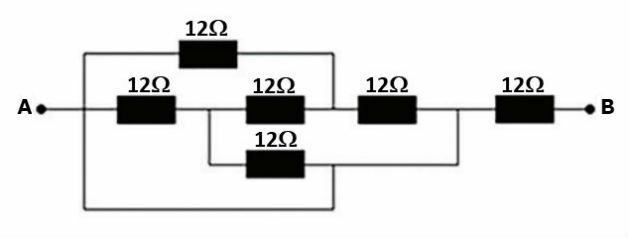
a) 0
b) 12
c) 24
d) 36

See Answer

Naming each node in the circuit, we have the following configuration:

As the ends of the five resistors marked are connected to point AA, therefore, they are short-circuited. We then have a single resistor whose terminals are connected to points AB.

Therefore, the equivalent resistance of the circuit is equal to 12 Ω.

Alternative: b) 12