THE strength weight (P) is a type of force that acts in the vertical direction under the pull of Earth's gravitation.
In other words, it is the force that exists on all bodies, being exerted on them through the Earth's gravitational field.
Weight Strength Formula
To calculate the weight force, use the following formula:
P = m. g (in module)
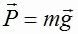(in vector)
Where,
P: strength weight (N)
m: mass (kg)
g: gravity acceleration (m/s2)
Remember that force is a vector, so it is indicated by an arrow above the letter. Vectors have modulus (strength of the force exerted), direction (the line along which it acts) and direction (the side of the line on which the force was exerted).
In standard gravity, that is, at a location where the gravitational acceleration is 9.8 m/s2, one kilogram force (1kgf) is the weight of a body of one kilogram of mass:
1kgf = 9.8N
Did you know?
The weight of the bodies can vary according to the gravity of the place. That is, the weight of a body is different on planet Earth, with a gravity of 9.8m/s
2, and on Mars, where gravity is 3.724m/s2.Therefore, when we say “I weigh 60 kg”, we are using an incorrect expression according to physics.
The correct one would be “I have a mass of 60Kg”. This is because while the weight of a body varies according to gravity, the mass never varies, that is, it is constant.
To learn more, read also: gravitational force.
Examples
Below are three examples of how to calculate strength weight:
1. What is the weight of a body of mass 30 kg on the surface of Mars, where gravity is equal to 3.724m/s2?
P = m. g
P = 30. 3,724
P = 111.72 N
2. Calculate the weight of a 50 kg object on the earth's surface where gravity is 9.8m/s2?
P = m. g
P = 50. 9,8
P = 490 N
3. What is the weight of a 70 kg person on the moon? Consider the gravity on the moon to be 1.6m/s2.
P = m. g
P = 70. 1,6
P = 112 N
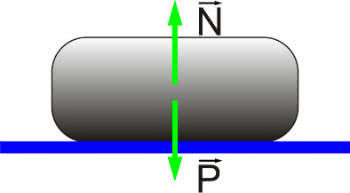
Normal force
In addition to weight strength, we have the normal force which also acts in the vertical direction in a straight plane. Thus, the normal force will be of the same intensity as the weight force, however, in the opposite direction.
For a better understanding, see the figure below:
Entrance Exam Exercises with Feedback
1. (PUC-MG) Suppose your mass is 55 kg. When you step on a pharmacy scale to find your weight, the pointer will indicate: (consider g=10m/s2)
a) 55 kg
b) 55 N
c) 5.5 kg
d) 550 N
e) 5,500 N
Alternative
2. (ENEM) The weight of a body is a physical quantity:
a) which does not vary with the location of the body
b) whose unit is measured in kilograms
c) characterized by the amount of matter that the body contains
d) which measures the strength of the support reaction force
e) whose intensity is the product of the mass of the body and the acceleration of local gravity.
Alternative and
3. (Unitins-TO) Check the correct proposition:
a) the mass of a body on Earth is less than on the Moon
b) weight measures the inertia of a body
c) Weight and mass are synonymous
d) The mass of a body on Earth is greater than on the Moon
e) The jet propulsion system works based on the principle of action and reaction.
Alternative and
4. (UNIMEP-SP) An astronaut in full suit has a mass of 120 kg. When being taken to the Moon, where the acceleration of gravity is equal to 1.6m/s2, its mass and weight will be, respectively:
a) 75 kg and 120 N
b) 120 kg and 192 N
c) 192 kg and 192 N
d) 120 kg and 120 N
e) 75 kg and 192 N
Alternative b
5. (UFV-MG) An astronaut takes a box from Earth to the Moon. We can say that the effort he will make to carry the box on the Moon will be:
a) greater than on Earth, as the box's mass will decrease and its weight will increase.
b) greater than on Earth, as the box's mass will remain constant and its weight will increase.
c) smaller than on Earth, as the box's mass will decrease and its weight will remain constant.
d) smaller than on Earth, as the box's mass will increase and its weight will decrease.
e) smaller than on Earth, as the box's mass will remain constant and its weight will decrease.
Alternative and
Want to know more? Continue your research by reading the texts:
- Strength
- Gravity
- Newton's Laws
- Newton's Laws - Exercises
- Gravity Acceleration
- Pulleys
- Physics Formulas
