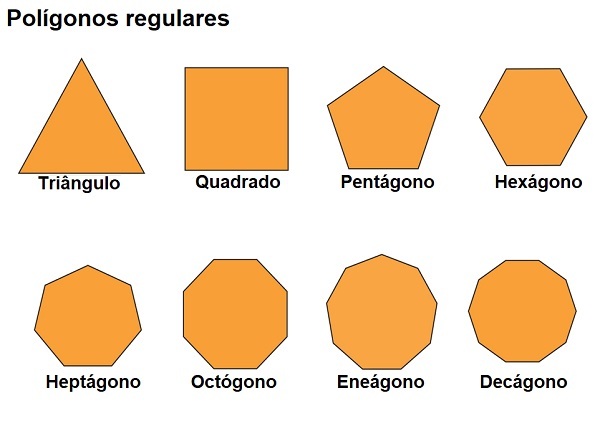
THE polygon classification is used to name them. For example, when the polygon it has exactly three angles, it is called a triangle; when it has four angles, it is called a quadrilateral. Above four sides, polygons are named as pentagons, hexagons, and so on.
It is possible to classify the polygons also according to the measure from its sides and also from its angles. With respect to sides, a polygon can be regular, when it has sides and angles congruent, or irregular. As for angles, it can be classified as convex, when all its angles are less than 180º, or concave (non-convex), when it has at least one angle greater than 180º.
Read too: Triangle classification - criteria and nomenclature
polygon classification
A polygon can be classified according to its characteristics. One is the number of sides or angles. In addition to this classification, a polygon can be considered regular or irregular, according to the measure of its angles and the congruence or not of its sides. A third classification of polygons takes into account the size of their interior angles. When one of them is an angle greater than 180°, this polygon is known as non-convex or concave.

As for the number of sides or angles
To recognize and name a polygon, we take into account the number of sides or the number of angles it has, which are even equal. Polygons with fewer sides are the triangle (three angles) and the quadrilateral (four sides). From a five-sided polygon, there is a pattern in the construction of the names of these polygons: we present the quantities with the Greek prefix corresponding to the number of sides plus the suffix -gono.
The use of quantities in Greek is quite common in mathematics and chemistry. The most common prefixes are:
Penta → five
Hexa → six
Hepta → seven
Octa → eight
Enea → nine
Deca → ten
Hendeca or undeca → eleven
Dodeca → twelve
Icosa→ twenty
Thus, when we add the number of sides in Greek with the ending -gono (which means angle), we will find:
Pentagon → 5-sided polygon
Hexagon → 6-sided polygon
Heptagon → 7-sided polygon
Octagon → 8-sided polygon
Enneagon → 9-sided polygon
Decagon → 10-sided polygon
Undecagon or hendecagon → 11-sided polygon
Dodecagon → 12-sided polygon
Icosagon → 20-sided polygon

The two-dimensional universe is often confused with the three-dimensional, which does not use the gono ending (which mentions the angle), but the -hedron termination (which mentions the faces), what happens with the Geometric solids, such as icosahedron, dodecahedron, among others, which are three-dimensional and known as polyhedra.
See too: Differences between flat and spatial figures
Do not stop now... There's more after the advertising ;)
Regular and Irregular Polygon
A polygon can be classified as regular when he has all the congruent angles and sides. Being congruent means having the same measure. The equilateral triangle and the square are examples. When at least one side is different, the polygon is irregular.
The term equilateral is used in reference to equal sides. The same reasoning applies to angles, with the term equiangular.
Convex and non-convex polygons
There are several ways to explain what a convex polygon and a non-convex polygon. Geometrically, we can say that a polygon is convex when, by choosing any two points A and B, the ifstraight segment that unites these two points is contained in the polygon. Otherwise, that is, if there are at least two points contained in the polygon whose line segment connects them is not contained in the polygon, he is known as not convex or concave.
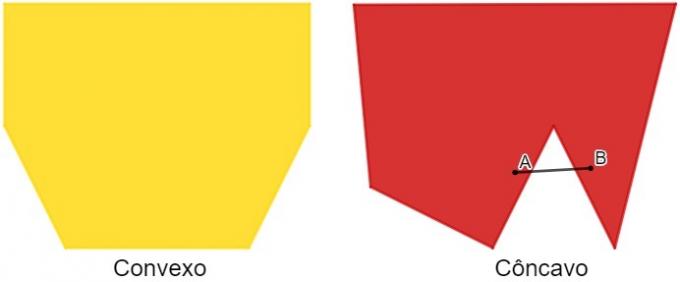
A very easy way to identify is by looking at the polygon's interior angles. When it has an angle greater than 180°, it will therefore be a non-convex polygon.
Also access: Parallelograms - polygons that have parallel opposite sides
solved exercises
Question 1 - Analyzing the polygon below, we can classify it as:

A) hexagon, convex and regular.
B) hexagon, non-convex and irregular.
C) pentagon, convex and regular.
D) pentagon, concave and irregular.
E) quadrilateral, convex and regular.
Resolution
Alternative D. Analyzing the figure, we can say that it has five sides, so it is a pentagon. It has an angle AÊD greater than 180º, which makes it also concave, that is, not convex. Finally, the angles aren't all the same, which makes it irregular, so it's an irregular concave pentagon.
Question 2 - About the polygon classifications, judge the following statements:
I – Every triangle is convex.
II – We define a regular polygon as one that has all congruent angles.
III – Every convex polygon is regular.
We can say that:
A) only I is true.
B) only II is true.
C) only III is true.
D) only I and II are true.
E) only II and II are true.
Resolution
Alternative A.
→ 1st step: judge the statements.
I - Every triangle is convex.
True, as the triangle's internal angles are always less than 180°, as the sum of the three angles equals 180°.
II - We define a regular polygon that has all congruent angles.
False as not only the angles but also the sides need to be congruent. The rectangle is an example of a non-regular polygon that has congruent angles.
III - Every convex polygon is regular.
False. To be convex, it just needs to have angles smaller than 180º, which doesn't mean that it needs to have congruent sides and angles.
→ 2nd step: analyze the alternatives.
Only I is true.
By Raul Rodrigues de Oliveira
Maths teacher