Hydrostatics is a branch of physics that studies the characteristics of fluids at rest. In particular, it establishes relationships with the pressure exerted on bodies immersed in fluids such as atmospheric air and water.
Lookalso:Important concepts for the study of hydrostatics
What is Hydrostatics?
THE Hydrostatics is an area of Physics that studies the properties of fluids at rest. Among the physical properties of fluids, we can highlight the most important: density,pressure and strength of buoyancy. We understand as fluids substances capable of assuming the shape of their container, changing their shape under the action of some external force.
THE density is an important parameter, as this property measures the amount of matter a fluid has in a given space. According to the International System of Units (SI), the density of a fluid is measured in kilogramspersubwaycubic (kg/m³).
THE pressurehydrostatic measures the force per unit area that a fluid at rest is capable of exerting against a surface. The greater the depth of a body immersed in a fluid, the greater the pressure exerted on it. The pressure unit in the SI is the
paschal (Pa), which is equivalent to the pressure of 1 newton per square meter (N/m²).O buoyancy, in turn, it is the force that every fluid exerts on the bodies immersed in it. The buoyant force is responsible for expelling gas bubbles from carbonated beverages. In addition, it makes a cork, a ship or an ice cube float on water. The buoyant force is described by the Archimedes' Theorem, and your unit is the newton (N).
hydrostatic pressure
THE pressurehydrostatic is the pressure exerted by a column of fluid at rest. To calculate the modulus of the hydrostatic pressure exerted by a fluid, we use the principlefundamentalgivesHydrostatics:
The pressure difference between two points in a fluid is determined by the product of its density, the local gravity modulus, and the height difference between these points. |

The fundamental principle of Hydrostatics states that the greater the depth of a fluid, the more pressure it will exert.
We can translate the fundamental principle of hydrodynamics in the following equation:
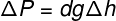
ΔP – pressure difference (Pa)
d – fluid density (kg/m³)
oh – height difference between fluid points (m)
A careful analysis of the principle presented above allows us to conclude that:
Two points that lie at the same height in a fluid they will have the same pressure;
all fluid in balance presents its free surface arranged in the direction horizontal;
The pressure in a fluid increases with its depth.
→ Pascal's theorem and pressure
According to Pascal's theorem, every pressure increase over an ideal fluid, that is, a fluid non-compressible, continuous and withoutviscosity, it is transmitted homogeneously throughout its volume. One of the applications of Pascal's principle is in the operation of hydraulic presses and pistons.

F – applied force (N)
THE – area of application of force (m²)
Hydrostatics and density
THE density it is one of the most important properties of a fluid. Through it, it is possible to determine the amount of matter that constitutes a fluid in a given volume. The density definition is shown below:

d – density (kg/m³)
m – mass (kg)
V – volume (m³)
The density of a fluid is measured based on the density of pure water, whose modulus is 1.0 kilogrampersubwaycubic. There are several density units commonly used in the study of hydrostatics. Check out some of them in the figure below and take the opportunity to learn how to perform unit conversions when necessary:

Equivalence between the main density units.
Lookalso: Differences between density and specific mass
Buoyancy and Hydrostatics
When inserted into a fluid, a body occupies part of the space previously occupied by the fluid itself. Thus, the fluid will exert on this object a force directed upwards of a magnitude equal to the weight of the fluid that was displaced due to the insertion of the body inside it.
The definition presented above was developed by ArchimedesinSyracuse, an important mathematician, inventor and physicist Greek. Your original statement is presented below:
"Every body immersed in a fluid at rest undergoes, on the part of the fluid, an upward vertical force, the intensity of which is equal to the weight of the fluid displaced by the body." |
The modulus of the buoyant force can be calculated using the following definition:

The thrust exerted depends on the volume (V) of fluid displaced by the insertion of a body, fluid density and local gravity.
The buoyancy exerted by a fluid noit will depend, therefore, of the Weightofbody or yours density, but yes of densityoffluid, gives gravitylocal It's from volumeinfluiddisplaced, which, by the way, is equal to the volume of the body portion that is immersed at the fluid.
Although buoyancy is not determined by body density, through the relationship between body and fluid densities, it is possible to know if the body will float, will remaininbalance or will submerge. Watch:
if the body is moredense that the bottom, it will tend to sink;
If the body has a density next that of the fluid, it will tend to remain in balance;
If the body has density smaller than the density of the fluid, it will tend to fluctuate.
apparent weight
Weightapparent is the result of forces Weight and buoyancy which act on a body embedded in a fluid. When immersed in fluid, the body will appear more "Light" than it really is. This is because the buoyant force acts on this body in the direction vertical, always pointing forup.
O Weightapparent can be calculated as follows:

PAP – apparent weight (N)
P – body weight (N)
AND – thrust on the body (N)
Hydrostatic Formulas
Check below some of the main formulas used in the study of Hydrostatics.
→ Fundamental Principle of Hydrostatics
The fundamental principle of Hydrostatics makes it possible to calculate the pressure difference between two points of different heights within an ideal fluid.

→ Density
Density measures the amount of matter contained in a fluid or a body per unit of space occupied by it:

→ Pascal's Theorem
Pascal's theorem indicates that the increase in pressure exerted on an equilibrium fluid is distributed over it in an integral and homogeneous way:

→ Buoyancy
Buoyancy is the force exerted by a fluid that has been displaced from its position as a result of the insertion of a body. The buoyancy depends on the volume of fluid displaced, local gravity and fluid density:
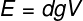
→ apparent weight
Apparent weight is the resulting force on a body inserted fully or partially into a fluid:
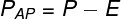
Hydrostatic Exercises
1. Determine the pressure difference between two points located 10 m and 20 m deep in a brackish water lake with a density equal to 1200 kg/m³.
adopt:
g = 10 m/s²
Resolution
To calculate the pressure difference between the two points mentioned in the exercise, we will use the fundamental principle of Hydrostatics:
'
In this way, we will have the following calculation:

By Me. Rafael Helerbrock
