THE binding energy is involved in the breakorin training of one or more bonds between atoms of a molecule. The hydrogen gas molecule, for example, has a single bond (sigma) between the atoms involved:

Hydrogen gas structural formula
For this molecule to have originated, the single bond between its atoms was formed. When this molecule participates in a chemical reaction with chlorine gas (Cl2), for example, for the formation of hydrochloric acid (HCl), the single bonds present in H2 and in the Cl2 must be broken with the consequent formation of a single bond in the HCl.
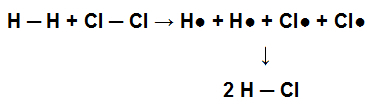
Representation of the breaking of single bonds in the reagent and formation of a single bond in the product
Atoms bond together to achieve their electronic stability, that is, they move from a higher energy situation to a lower energy situation. Thus, we can say that when the bond between atoms is formed, energy is released; therefore, consequently, its breakdown depends on energy absorption.
If we understand that the breakup (breaking) of a chemical bond occurs when it is supplied to it
an amount of energy (xKcal), we concluded that this is a process endothermic. In contrast, forming a bond will involve the release gives same amount of energy (-xkcal), being, then, a process exothermic.Like chemical reactions are classified into endothermic or exothermic, we can use knowledge of the binding energies of the molecules of reactants and products to determine the change in energy (ΔH) of the chemical process and then classify it.
For example, see the equation below:
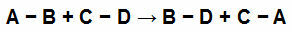
Chemical Bonds in Participants in a Chemical Equation
We have simple links in each of the reaction participants. They have the following values:
[A-B] = 50 Kcal
[CD] = 100 Kcal
[B-D] = 80 Kcal
[B.C] = 230 Kcal
With the values above, we can calculate the energy involved in breaking the bonds of reactants and forming the bonds of products as follows:
In reagents
50 Kcal to break the AB bond and 100 Kcal to break CD bond, that is, in the reagent, 150 Kcal will be used to break the bonds.
In products
80 Kcal to form BD bond and 230 Kcal to form AC bond, that is, 310 Kcal will be released in the product in the formation of bonds
With the values of the energies involved in the reactants and products, it is possible to know whether the reaction has absorbed or released more energy just by subtracting the energy used in the disruption from the energy released in the formation:
ΔH = Energy of reagents - Energy of products
ΔH = 230 - 310
ΔH = -80 Kcal
Do not stop now... There's more after the advertising ;)
As the reaction has more energy release than absorption, negative ΔH is therefore exothermic.
NOTE: If the participant's stoichiometric coefficient is different from 1, we must multiply the energy value of the connection by the coefficient, for example:
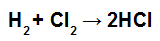
HCl formation equation
Since the coefficient of HCl is 2, we must multiply the value of the binding of HCl by 2.
Now follow the resolution of an exercise on binding energy in a chemical reaction:
Example: Given the following binding energies, in kilojoules per mole of bonds (absolute values):
H − H = 436
N ≡ N = 945.6
N − H = 391
Calculate the heat (in kilojoules per mol of NH3(g)) involved in the reaction represented by:
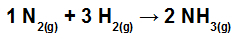
Chemical bonds in the NH formation reaction3
Resolution:
The first step in solving this exercise is to rewrite the equation provided demonstrating the chemical bonds present in each of the molecules:
No N2: we have a triple bond (because the N, family of Nitrogen, must make three bonds because it has five electrons in the valence shell);
at H2: We have a single bond (because the H must make only one bond because it has only one electron in the valence shell);
in NH3: We have the presence of three simple bonds (because each H needs a bond, and the N, three bonds).
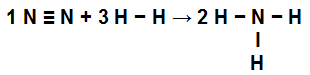
Equation demonstrating the links in NH formation3
As the exercise provided the values for each binding, the first step is to determine the binding energy related to each of the participants:
- To the N2
We have 1 mole in N2in the equation and, to break your connection, we need 945.4 KJ, therefore:
1.945,4 = 945.4 KJ
- To the H2
We have 3 mol in H2in the equation and, to break your connection, we need 436 KJ, therefore:
3.346 = 1038 KJ
- To NH3
We have 2 mol of NH3in the equation, but there is three times the N-H bond, so let's multiply the amount of energy involved to form that bond by 2 and by 3:
2.3.391 = 2346 KJ
Finally, we can determine the heat involved in the reaction by subtracting the energy used to break the reactant from the energy released in forming the product:
ΔH = energy in reactants - energy in products
ΔH = (945.4 + 1038) - 2346
ΔH = 1983.4 - 2346
ΔH = - 362.6 KJ per mol of NH3(g)
As the variation was negative, it means that the energy released in the formation of bonds in the products was greater than the energy absorbed in breaking the bonds of the reactants, therefore, the reaction is exothermic.
By Me. Diogo Lopes Dias

