Transformation equations are fundamental in the study of relativity, as they relate the coordinates of the movement of two references that move in relation to each other, that is, they relate position, velocity and time in the two referential. The Italian physicist Galileo Galilei deduced, in the 16th century, what we call Galileo's transformation equations, and to understand them let's understand consider the figure below in which we have two inertial frames, S' and S, and the frame S' moves with velocity v in relation to referential S.
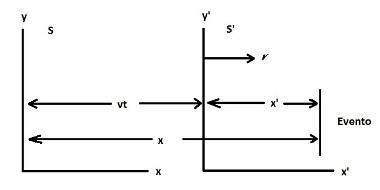
Two inertial reference systems, where S' moves with respect to S, and moves away with velocity v
If we place an observer in the S frame, for him the space-time coordinates of a given event will be x, y, z, t, on the other hand an observer in the S frame. it will have for the same event x', y', z', t' coordinates, and the y and z coordinates will remain constant, not being influenced by the movement, so we can say what:
y = y' and that z = z'
Galileo's transformation equations, according to the figure above, are:
x' = x - vt
t = t'
These equations are valid for velocities (v) much lower than the speed of light (c), that is, for v << c, because when v tends to approach c, these equations start to disagree with experimental results, for these cases we should use the Lorentz transformation equations.
Do not stop now... There's more after the advertising ;)
Hendrik Antoon Lorentz was a great Dutch physicist responsible for deducing fundamental equations for the study of relativity, the so-called Lorentz equations (also known as Lorentz transforms) which are as follows:
x' =ϒ(x – vt)
y' = y
z' = z
t' = ϒ(t - vx)
c²
These equations are valid for all speeds, note that if v is much less than c (v << c), they will reduce to Galileo's equations, this shows a more general characteristic of relativity in relation to physics classic. The ϒ factor is called the Lorentz factor and can be calculated using the equation below:
ϒ = 1
[ 1 - (v/c) ²]1/2
Lorentz's equations can be rewritten by swapping the x' and x coordinates, as well as t' and t, and also by inverting the velocity sign (v), thus:
x = ϒ(x' + vt')
t = ϒ(t'+vx')
c²
By Paulo Silva
Graduated in Physics
Would you like to reference this text in a school or academic work? Look:
SILVA, Paulo Soares da. "Lorentz Transformation"; Brazil School. Available in: https://brasilescola.uol.com.br/fisica/transformacao-lorentz.htm. Accessed on June 27, 2021.