What is osmoscopy?

osmoscopy is joint ownership (the others are tonoscopy, ebullioscopy and cryoscopy) which studies the occurrence of osmosis between two solutions of different concentrations, one of which is more concentrated than the other.

Note: Colligative properties occur when a non-volatile solute is added to a solvent.

Such as osmoscopy study osmosis, it is essential to know What is this phenomenon. For this, we will use the solutions below, which are separated by a semi-permeable membrane:

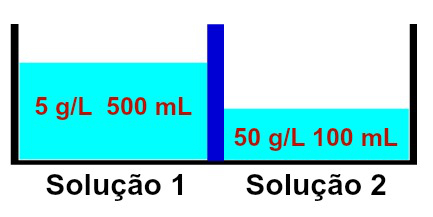
Representation of solutions that have different concentrations

It is observed that solution 1 contains a concentration of 5 g/L and a volume of 500 mL, while the solution 2 has a concentration of 50 g/L and a volume of 100 mL, separated by a membrane semipermeable. Solution 2 is more concentrated than solution 1 and therefore osmosis must occur between them.

Osmosis must necessarily happen from solution 1 to solution 2, since solution 1 is less concentrated. During this occurrence, part of the solvent crosses the semi-permeable membrane, making the solution volume 2 increase and the volume of solution 1 decrease, until both solutions start to have the same concentration, that is, isotony.

Modification in height of solutions 1 and 2 due to the occurrence of osmosis

Note: Isotonic media are those whose concentration is equal.

According to the osmoscopy, osmosis takes place because the maximum vapor pressure of the solvent in the less concentrated solution is greater than that of the solvent in the more concentrated solution. Now, if we want to prevent the occurrence of osmosis, just exert pressure on the most concentrated solution:

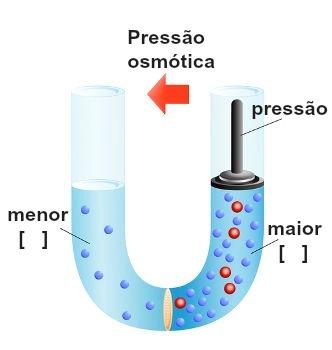
Representation of the execution of pressure on the most concentrated solution

This pressure, which is exerted on the most concentrated solution to block or even reverse osmosis, is called osmotic pressure and is represented by the symbol π. It should be directly proportional to the concentration of the solution.

Possible interpretations of osmotic pressure

According to the conclusions of the osmoscopy, each solution has an osmotic pressure, since this is related to the concentration, a characteristic present in every solution.

Do not stop now... There's more after the advertising ;)

When comparing one medium or one solution to another, we can use the following terms:

• Hypertonic: when one medium presents an osmotic pressure greater than the other;

• hypotonic: when one medium has an osmotic pressure lower than the other;

• Isotonic: when the two media or solutions have the same osmotic pressure.

Thus, when comparing the osmotic pressures of two solutions A and B, represented by π_THE and π_B, we can say that :

• If the osmotic pressure of A and B are equal, the means or solutions will be isotonic:

π_THE = π_B

• If the osmotic pressure of A is greater than the osmotic pressure of B, medium A will be hypertonic in relation to B:

π_THE> π_B

• If the osmotic pressure of B is less than the osmotic pressure of A, medium B will be hypotonic in relation to B:

π_B< π_THE

Formula for calculating osmotic pressure

π = M.R.T

In this formula:

• π = is the osmotic pressure

• M = is the concentration in mol/L

• R = is the general gas constant (0.082 for pressure in atm; 62.3 for pressure in mmHg)

• T = temperature in Kelvin

As the concentration in mol/L has a particular formula, as shown below:

M = m₁
M₁.V

We can replace it in the osmotic pressure formula:

π = m₁.R.T
M₁.V

Note: If the solute present in the solution is ionic, we must use the Van't Hoff correction factor (i) in the expression of the osmotic pressure calculation:

π = M.R.T.i

Example of osmotic pressure calculation

Example: (UF-PA) A solution containing 2 mg of a new antibiotic, in 10 mL of water, at 25 ºC, produces an osmotic pressure of 0.298 mmHg. So, the molecular mass of this antibiotic is, approximately:

a) 3000

b) 5200

c) 7500

d) 12500

e) 15300

The data provided by the exercise were:

• π = 0.298 mmHg

• T = 25 ^OC or 298 K (after adding with 273)

• m₁ = 2 mg or 0.002 g (after dividing by 1000)

• V = 10 mL or 0.01 L (after dividing by 1000)

• R = 62.3 mmHg

To solve this exercise, just apply the available data in the expression for calculating the osmotic pressure, as follows:

π = m₁.R.T
M₁.V

0,298 = 0,002.62,3.298
M₁.0,01

0.298.M₁.0,01 = 37,1308
0.00298.M₁ = 37,1308

M₁ = 37,1308
0,00298

M₁ = 12460 u

By Me. Diogo Lopes Dias

Would you like to reference this text in a school or academic work? Look:

DAYS, Diogo Lopes. "What is osmoscopy?"; Brazil School. Available in: https://brasilescola.uol.com.br/o-que-e/quimica/o-que-e-osmoscopia.htm. Accessed on June 28, 2021.